To most people, maths is just something we learned in order to avoid being ripped off. To some, maths is an essential tool, helpful in modelling plague outbreaks or cracking encryption ciphers.
However, for an elite few, maths is simply a parallel universe and they are its explorers.
Today let us discuss what I consider perhaps the most beautiful discovery to date. But first, some introductions…
Part 1: Consider, to start, the circle
If you have a wheel a metre across, it will roll out about 3.14159…metres each revolution. This number, which we call π turns out to be some sort of fundamental property of ‘space’.

The Greeks were not very happy about the ‘messiness’ of this number. They preferred numbers that could be expressed as fractions – while 22/7 was close to π, it was not exact and they lost a lot of sleep trying to find a neat way to write π.
Mathematicians have since grudgingly accepted that it cannot be written as a fraction, and indeed it cannot be written down at all because it has no ‘pattern’ and never ends, those digits just keep coming at (almost) random! Here are the first 100…
3.1415926535897932384626433832795028841971693993751058209749445923078164062862089986280348253421170679 ….

The wife of pi…
Understandably, they decided to call this sort of number ‘irrational‘.
Part 2: Consider now, ‘powers’
Mathematicians may work tirelessly on some very pointless looking things, however, they are still fairly lazy when it comes to writing stuff down. They like shorthand. So rather than writing 3+3+3+3+3 they invented ‘multiplication’, giving them 5×3.
Likewise, rather than writing 3x3x3x3x3 they invented ‘powers’, so they could write 35.
Of course they then realized these tricks could be extended past ‘whole’ numbers. 2.5×3 is 7.5. But what about 32.5?
It works of course, the answer turns out to be about 15.59 plus change.
But what does it mean? 32.5 is three, times by itself, 2.5 times! or 3x3x30.5. What on earth is that?
Well it turns out, when you ponder this (maybe I should say, if you ponder this), that 30.5 is the same as √3. So ‘root three’ is three times itself half a time…
[pregnant pause]
Ok, let’s look at it another way
Consider, for example 32x33, which is the same as (3×3)x(3x3x3) which is the same as 3x3x3x3x3 which is the same as 35 , so 3(2+3).
So using that logic…
3 = 31 = 3(0.5+0.5) = 30.5 x 30.5
And what times itself is equal to 3? Well √3! So 30.5 is √3…
It makes sense now, and we can even get used to saying things like 31.9 x 30.1 = 9.
Of course, these fractional powers also commonly yield those ‘messy numbers’, so abhorred by the Greeks. √3 is, roughly:
1.73205080756887729352744634150587236694280525381038062805580…
The logic follows through for negative numbers. 3-2 is just 1/32 which is 1/9.
Part 3. Now consider ‘e’
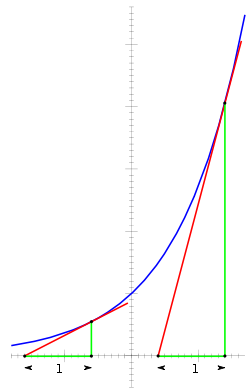
y=e^x. The slope is always the same as the value! This has the interesting effect that the tangent to the line always intercepts the y axis precisely 1 unit back…
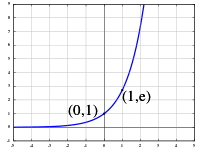
y=e^x
Here is a third sort of messy number, one which the Greeks are probably glad they missed. We have Leonhard Euler to thank for discovering this one.
He noted there was a number ‘e‘ giving an equation of the form y=ex (see the graphs pictured), where the slope of the curve is the same as the height of the curve at each point.
Strange and pointless sounding perhaps but pretty simple. So y=2x doesn’t work, y=3x doesn’t work, but by trial and error you can find a value for a that works, which is, roughly:
2.71828182845904523536028747135266249775724709369995…
It too has no pattern and no repeats so is also ‘irrational‘. This number has a whole book written about it, for those who are keen.
Part 4. Now consider ‘i’
The last piece of the puzzle now.
Consider the equation 3 + x = 0
Now solve for x. Seems pretty easy, but really you are cheating. There is no number that solves that equation. Really, to solve it you had to ‘invent’ the concept of a negative number.
Ok. Now consider the equation x2 + 1 = 0
Ah. Trickier! However it turns out that we can do the same trick; this time we simply invent another sort of number – the ‘imaginary’ number. Now if you’ve never heard about these numbers before, you may think I’m joking. Alas I am not. This is what mathematicians have been up to for the last few hundred years, just making stuff up as they go along.
So we define i as √-1, or a number, that when multiplied by itself, yields the more respectable -1.
Aside: Just as -1 is a number which, when multiplied by any negative number renders it decent (i.e. positive) once more.
So i2 + 1 = 0 and the equation is solved. It turns out mathematicians were suddenly able to solve loads of really tedious equations using this trick, which made their entire week.
So we have now got i! At last we are ready to put the puzzle together.
Simplicity emerges from the complex…
So, now I ask, what happens if you raise e to the power of i? What does it even mean? It means, e times itself √-1 times. Ouch. Nonsense surely?
Well it works out at roughly 0.540302306 + 0.841470985 i, which is a right mess, something they call, for fairly self-explanatory reasons, a complex number.
So now lets stick our old friend from the circle, π, in there and see what happens:
What is eiπ?
Surely an even bigger mess? I mean its all these messy irrational numbers combined with this home-made imaginary number…
Google can do it for you… and the answer is…..
-1.
So there you have it, all these messy numbers – π, e and non-integer powers combine with i and the answer pops out as -1.
Blows my mind.
—————–
For more info on whay the heck this is, look up Euler’s Identity!


 No small part of the doubling in life expectancy was due to vaccines.
No small part of the doubling in life expectancy was due to vaccines.